Summary (English)
This scientific paper investigates the relationship between renormalized volume, Polyakov anomaly, and orbifold Riemann surfaces in the context of holographic duality.
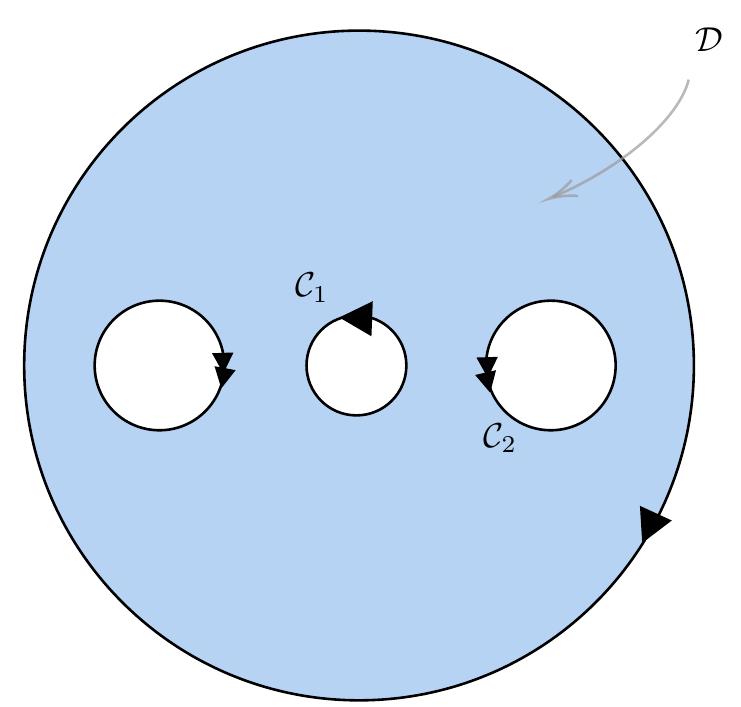
The authors study a function Sm for orbifold Riemann surfaces and prove its equivalence to the renormalized hyperbolic volume Vren of corresponding Schottky 3-orbifolds with lines of conical singularities reaching the conformal boundary.
This principle applies to both classical Liouville actions on Sg and Sg,n(∞).
The paper demonstrates that Vren acts as a Kähler potential for a specific combination of Weil–Petersson and Takhtajan–Zograf metrics in local index theorems for orbifold Riemann surfaces.
Furthermore, they show that Sm is a consistent height function with a unique hyperbolic solution, implying Vren also admits a Polyakov anomaly formula.
The method used to establish this equivalence could provide an alternative approach to deriving the renormalized Polyakov anomaly for Riemann surfaces with punctures (cusps).
The authors study a function Sm for orbifold Riemann surfaces and prove its equivalence to the renormalized hyperbolic volume Vren of corresponding Schottky 3-orbifolds with lines of conical singularities reaching the conformal boundary.
This principle applies to both classical Liouville actions on Sg and Sg,n(∞).
The paper demonstrates that Vren acts as a Kähler potential for a specific combination of Weil–Petersson and Takhtajan–Zograf metrics in local index theorems for orbifold Riemann surfaces.
Furthermore, they show that Sm is a consistent height function with a unique hyperbolic solution, implying Vren also admits a Polyakov anomaly formula.
The method used to establish this equivalence could provide an alternative approach to deriving the renormalized Polyakov anomaly for Riemann surfaces with punctures (cusps).
요약 (Korean)
이 과학 논문은 홀로그램 이중성의 맥락에서 재 정규화 된 부피, 폴리아 코프 이상 및 orbifold riemann 표면 사이의 관계를 조사합니다.
저자는 Orbifold Riemann 표면에 대한 기능 SM을 연구하고 Conformal 경계에 도달하는 원뿔형 특이점의 선을 갖는 상응하는 Schottky 3-orbifolds의 정규화 된 쌍곡 볼륨 VRE와 동등성을 입증합니다.
이 원칙은 SG 및 SG, N (∞)의 고전적인 liouville 행동에 적용됩니다.
이 논문은 vren이 Orbifold Riemann 표면에 대한 로컬 인덱스 정리에서 Weil -Petersson과 Takhtajan – Zograf 지표의 특정 조합에 대한 Kähler 잠재력으로 작용한다는 것을 보여줍니다.
또한, 그들은 SM이 고유 한 쌍곡선 솔루션을 갖는 일관된 높이 함수임을 보여줍니다.
VREN은 또한 폴리아코프 이상 공식을 인정한다는 것을 암시합니다.
이러한 동등성을 확립하는 데 사용 된 방법은 구멍 (cusps)을 갖는 리만 표면에 대한 재 정규화 된 폴리아 코프 이상을 도출하기위한 대안적인 접근법을 제공 할 수있다.
저자는 Orbifold Riemann 표면에 대한 기능 SM을 연구하고 Conformal 경계에 도달하는 원뿔형 특이점의 선을 갖는 상응하는 Schottky 3-orbifolds의 정규화 된 쌍곡 볼륨 VRE와 동등성을 입증합니다.
이 원칙은 SG 및 SG, N (∞)의 고전적인 liouville 행동에 적용됩니다.
이 논문은 vren이 Orbifold Riemann 표면에 대한 로컬 인덱스 정리에서 Weil -Petersson과 Takhtajan – Zograf 지표의 특정 조합에 대한 Kähler 잠재력으로 작용한다는 것을 보여줍니다.
또한, 그들은 SM이 고유 한 쌍곡선 솔루션을 갖는 일관된 높이 함수임을 보여줍니다.
VREN은 또한 폴리아코프 이상 공식을 인정한다는 것을 암시합니다.
이러한 동등성을 확립하는 데 사용 된 방법은 구멍 (cusps)을 갖는 리만 표면에 대한 재 정규화 된 폴리아 코프 이상을 도출하기위한 대안적인 접근법을 제공 할 수있다.
기술적 용어 설명 (Technical Terms)
추출된 기술 용어가 없습니다.
Excerpt (English Original)
Prepared for submission to JHEP Renormalized Volume, Polyakov Anomaly, and Orbifold Riemann Surfaces Hossein Mohammadia,b ,Ali Nasehc ,Behrad Taghavic aDepartment of Physics, Sharif University of Technology, P.O.
Box 11155-9161, Tehran, Iran2024 bResearch Center for High Energy Physics Department of Physics, Sharif University of Technology, P.O.Box 11155-9161, Tehran, IranDec cSchool of Particles and Accelerators, Institute for Research in Fundamental Sciences (IPM), P.O.
26 Box 19395-5531, Tehran, Iran E-mail: hossein_mohammadi@physics.sharif.edu, naseh,btaghavi@ipm.ir Abstract: In [1], two of the authors studied the function Sm = Sm −π Pni=1(mi − 1 ) log hi for orbifold Riemann surfaces of signature (g; m1, …, mne; np) on the generalized mi Schottky space Sg,n(m).
In this paper, we prove the holographic duality between Sm and[hep-th] the renormalized hyperbolic volume Vren of the corresponding Schottky 3-orbifolds with lines of conical singularity that reach the conformal boundary.
In case of the classical Liouville action on Sg and Sg,n(∞), the holography principle was proved in [2] and [3], respectively.
Our result implies that Vren acts as a Kähler potential for a particular combination of the Weil–Petersson and Takhtajan–Zograf metrics that appears in the local index theorem for orbifold Riemann surfaces [4].
Moreover, we demonstrate that under the conformal transformations, the change of function Sm is equivalent to the Polyakov anomaly, which indicates that the function Sm is a consistent height function with a unique hyperbolic solution.
Consequently, the associated renormalized hyperbolic volume Vren also admits a Polyakov anomaly formula.
The method we used to establish this equivalence may provide an alternative approach to derive the renormalized Polyakov anomaly for Riemann surfacesarXiv:2412.19137v1 with punctures (cusps), as described in [5].
Contents 1 Introduction 1 2 Renormalized Volume and Holography Principle 3 3 Renormalized Volume and Polyakov Anomaly 13 4 Conclusion 18 A Asymptotic form of φ near punctures and conical points 21…
Box 11155-9161, Tehran, Iran2024 bResearch Center for High Energy Physics Department of Physics, Sharif University of Technology, P.O.Box 11155-9161, Tehran, IranDec cSchool of Particles and Accelerators, Institute for Research in Fundamental Sciences (IPM), P.O.
26 Box 19395-5531, Tehran, Iran E-mail: hossein_mohammadi@physics.sharif.edu, naseh,btaghavi@ipm.ir Abstract: In [1], two of the authors studied the function Sm = Sm −π Pni=1(mi − 1 ) log hi for orbifold Riemann surfaces of signature (g; m1, …, mne; np) on the generalized mi Schottky space Sg,n(m).
In this paper, we prove the holographic duality between Sm and[hep-th] the renormalized hyperbolic volume Vren of the corresponding Schottky 3-orbifolds with lines of conical singularity that reach the conformal boundary.
In case of the classical Liouville action on Sg and Sg,n(∞), the holography principle was proved in [2] and [3], respectively.
Our result implies that Vren acts as a Kähler potential for a particular combination of the Weil–Petersson and Takhtajan–Zograf metrics that appears in the local index theorem for orbifold Riemann surfaces [4].
Moreover, we demonstrate that under the conformal transformations, the change of function Sm is equivalent to the Polyakov anomaly, which indicates that the function Sm is a consistent height function with a unique hyperbolic solution.
Consequently, the associated renormalized hyperbolic volume Vren also admits a Polyakov anomaly formula.
The method we used to establish this equivalence may provide an alternative approach to derive the renormalized Polyakov anomaly for Riemann surfacesarXiv:2412.19137v1 with punctures (cusps), as described in [5].
Contents 1 Introduction 1 2 Renormalized Volume and Holography Principle 3 3 Renormalized Volume and Polyakov Anomaly 13 4 Conclusion 18 A Asymptotic form of φ near punctures and conical points 21…
발췌문 (Korean Translation)
JHEP 재 정규화 된 부피, 폴리아 코프 이상 및 Orbifold Riemann Surfaces Hossein Mohammadia, B, Ali Nasehc, Behrad Taghavic Adepartment, Sharif University of Technology, P.O.
Box 11155-9161, Tehran, IRAN2024 고 에너지 물리학 부서의 Bresearch Center, Sharif University of Technology, P.O.
Box 11155-9161, Tehran, Irandec Cschool of Fundamental Sciences (IPM), P.O.
26 Box 19395-5531, Tehran, Iran 이메일 : Hossein_mohammadi@physics.sharif.edu, naseh, btaghavi@ipm.ir 요약 : [1]에서 [1]에서 저자 중 두 명은 Sm = sm-π pni = 1 (mi-1) 로그에 대한 로그 (mi-1); …, mne; np) 일반화 된 Mi Schottky Space SG, N (M).
이 논문에서, 우리는 SM과 [HEP-TH] 사이의 홀로그램 이중성을 입증한다.
SG 및 SG, N (∞)에 대한 고전적인 liouville 행동의 경우, 홀로그래피 원리는 각각 [2] 및 [3]에서 입증되었습니다.
우리의 결과는 Vren이 Riemann 표면의 Orbifold 인덱스 정리에 나타나는 Weil -Petersson과 Takhtajan – Zograf 지표의 특정 조합에 대한 Kähler 잠재력으로 작용한다는 것을 의미합니다 [4].
또한, 우리는 함수 변환 하에서 기능 SM의 변화가 폴리아 코프 이상과 동일하다는 것을 증명한다.
결과적으로, 관련된 재 정규화 된 쌍곡 볼륨 VRE는 또한 폴리아코프 이상 공식을 인정한다.
우리 가이 동등성을 확립하는 데 사용 된 방법은 [5]에 설명 된 바와 같이, 펀처 (커프)를 갖는 Riemann Surfacesarxiv : 2412.19137v1에 대한 재 정규화 된 폴리아 코프 이상을 도출하기위한 대안적인 접근법을 제공 할 수있다.
목차 1 소개 1 2 재 정규화 된 부피 및 홀로그래피 원리 3 3 재 정규화 된 부피 및 폴리아 코프 이상 13 4 결론 18 구멍 및 원뿔형 지점 근처의 φ의 점근 형태 21 …
Box 11155-9161, Tehran, IRAN2024 고 에너지 물리학 부서의 Bresearch Center, Sharif University of Technology, P.O.
Box 11155-9161, Tehran, Irandec Cschool of Fundamental Sciences (IPM), P.O.
26 Box 19395-5531, Tehran, Iran 이메일 : Hossein_mohammadi@physics.sharif.edu, naseh, btaghavi@ipm.ir 요약 : [1]에서 [1]에서 저자 중 두 명은 Sm = sm-π pni = 1 (mi-1) 로그에 대한 로그 (mi-1); …, mne; np) 일반화 된 Mi Schottky Space SG, N (M).
이 논문에서, 우리는 SM과 [HEP-TH] 사이의 홀로그램 이중성을 입증한다.
SG 및 SG, N (∞)에 대한 고전적인 liouville 행동의 경우, 홀로그래피 원리는 각각 [2] 및 [3]에서 입증되었습니다.
우리의 결과는 Vren이 Riemann 표면의 Orbifold 인덱스 정리에 나타나는 Weil -Petersson과 Takhtajan – Zograf 지표의 특정 조합에 대한 Kähler 잠재력으로 작용한다는 것을 의미합니다 [4].
또한, 우리는 함수 변환 하에서 기능 SM의 변화가 폴리아 코프 이상과 동일하다는 것을 증명한다.
결과적으로, 관련된 재 정규화 된 쌍곡 볼륨 VRE는 또한 폴리아코프 이상 공식을 인정한다.
우리 가이 동등성을 확립하는 데 사용 된 방법은 [5]에 설명 된 바와 같이, 펀처 (커프)를 갖는 Riemann Surfacesarxiv : 2412.19137v1에 대한 재 정규화 된 폴리아 코프 이상을 도출하기위한 대안적인 접근법을 제공 할 수있다.
목차 1 소개 1 2 재 정규화 된 부피 및 홀로그래피 원리 3 3 재 정규화 된 부피 및 폴리아 코프 이상 13 4 결론 18 구멍 및 원뿔형 지점 근처의 φ의 점근 형태 21 …
출처: arXiv
답글 남기기