This study investigates the conceptual and mathematical foundations of reference frames in General Relativity using the fiber bundle framework.
It contrasts two paradigms: The View from Nowhere interprets frame representations as perspectives on an invariant equivalence class, while the View from Everywhere posits each frame representation as constituting reality itself, termed “Re(l)ality.
” The paper critically examines the philosophical and practical implications of these views, with a focus on reconciling theory with experimental practice.
It highlights the challenge of providing a perspicuous characterisation of ontology and discusses the role of reference frames in General Relativity.

이 연구는 일반 관절성 관점에서 참조 프레임의 개념적인 및 수학적인 기초를 탐색하고 꼭대기에서 보는 것과 모든 것이 그 자체로 구성되어 있는 ‘Re(l)ality’라는 개념을 비교하는 두 관점을 살펴본다.
이 연구는 이러한 관점의 필사심적 및 실용적 결과를 살펴보며, 이론과 실험적 연구에 대한 일치를 위해 기본 개념을 명확하게 설명하는 데 어려움을 나타낸다.
참조 프레임의 역할을 포함하여 일반 관절성에서 참조 프레임에 대한 해석은 이 연구에서 중점적으로 살펴본다.

1. 관점: 관점은 특정 시각에서 현실을 해석하는 방식을 말한다. 2. 관절성: 일반 관절성은 물리학의 원칙을 설명하기 위해 공간과 시간의 곡률적 변형을 고려하는 이론이다. 3. 동기: 동기는 관절성에서 필드 값들을 연결하여 관련성을 나타내는 것으로, 지역적인 물리 상태를 정의한다.

1. [1] Nicola Bamonti, “Re(l)ality: The View From Nowhere vs. The View From Everywhere”, arXiv:2412. 20520v1 [physics. hist-ph], 29 Dec 2024. Nicola Bamonti and Ricardo Gomes, “Reference frames in general relativity: from Kaluza–Klein to dynamical couplings”, arXiv:2312. 12381v1 [gr-qc], 2024

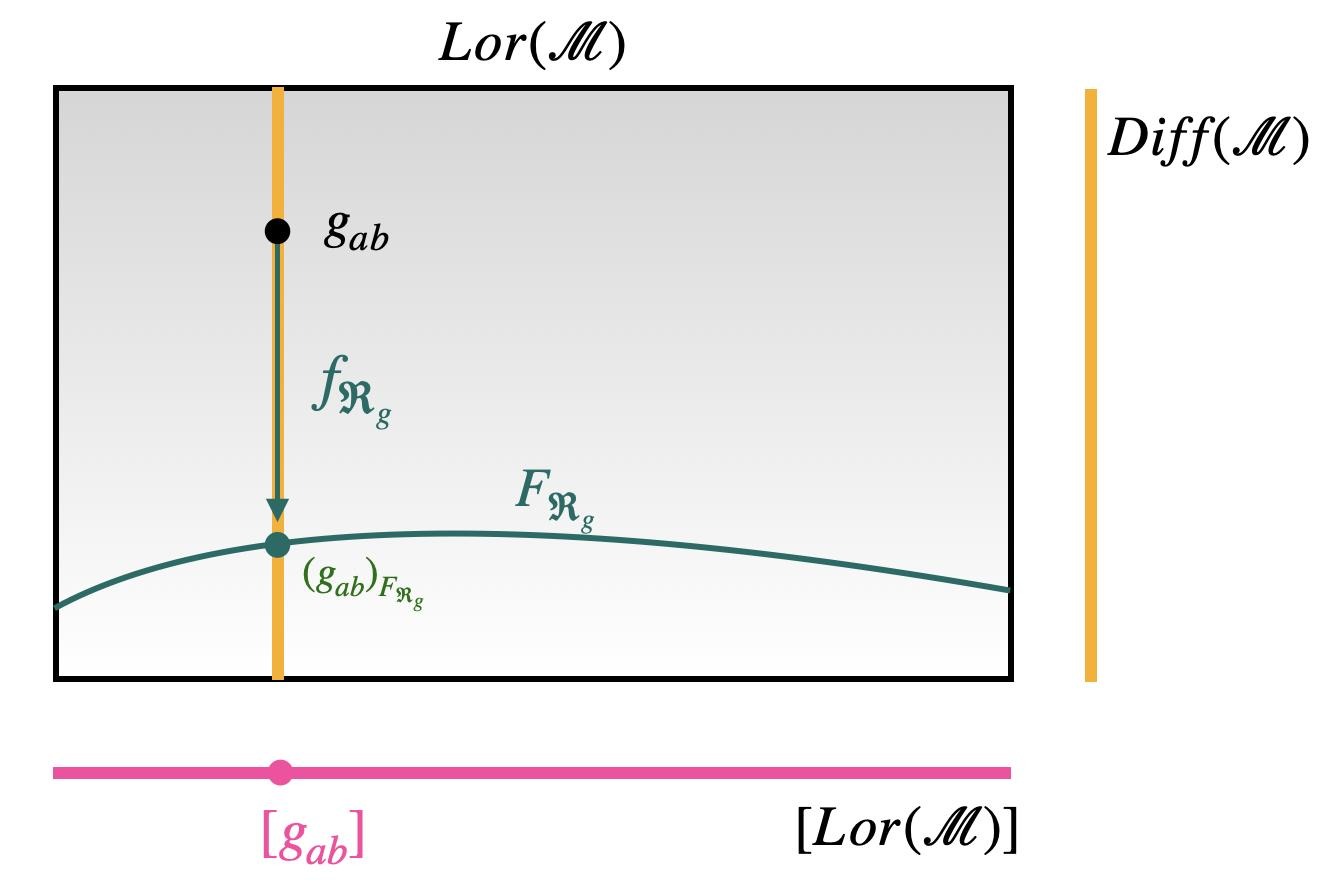
Re(l)ality: The View From Nowhere vs. The View From Everywhere Nicola Bamonti1,2 1Department of Philosophy, Scuola Normale Superiore, Piazza dei Cavalieri, 7, Pisa, 56126, Italy 2Department of Philosophy, University of Geneva, 5 rue de Candolle, 1211 Geneva 4, Switzerland Abstract Using the fiber bundle framework, this work investigates the conceptual and mathematical foundations of reference frames in General Relativity by contrasting two paradigms. The View from Nowhere interprets frame representations as perspectives on an invariant equivalence class, while the View from Everywhere posits each frame representation as constituting reality itself. This conception of reality is termed ”Relality.” The paper critically examines the philosophical and practical implications of these views, with a focus on reconciling theory with experimental practice. Central to the discussion is the challenge of providing a perspicuous characterisation of ontology. The View from Nowhere aligns with the so-called ‘sophisticated approach on symmetries’ and it complicates the empirical grounding of theoretical constructs. In contrast, the View from Everywhere offers a relational ontology that avoids the abstraction of equivalence classes. 1 arXiv:2412.20520v1 [physics.hist-ph] 29 Dec 2024 Contents 1 Introduction 3 2 The Bundle Formalism: A Gauge Perspective on Reference Frames 7 3 The View from Nowhere: Frame representations are perspectives on an equivalence class 12 4 The View from Everywhere: Frame representations are all that exist 17 5 Conclusion 23 2 1 Introduction In theories with gauge symmetries, such as General Relativity (GR), the term observables refers to quantities that remain invariant under transformations that leave the physical content of the theory unchanged. These transformations, known as gauge transformations, represent mathematical re- expressions of the theory’s underlying formalism. This paper explores how such observables are defined and interpreted. For a theory to achieve empirical validity, its gauge-invariant observables must correspond to measurable quantities, facilitating comparisons between theoretical predictions and experimental observations. This correspondence is a critical criterion for the acceptance of any scientific theory.1 All measurements are inherently local, as they rely on observations within specific regions of spacetime. Much of our experience involves measuring locally defined variables at particular spacetime points or within localized regions. As Gary and Giddings (2007) emphasise, “all we can truly observe is localised — we have no access to infinity.” In GR, gauge symmetries are expressed as diffeomorphisms. As a result, any quantity defined locally in spacetime does not remain invariant under these transformations, raising challenges for constructing local, gauge-invariant observables. In a diffeomorphism-invariant theory, variables can be broadly categorized into two types: (1) highly non-local quantities, which are defined across the entire spacetime, such as integrals over global regions, and (2) relational quantities, which are constructed by correlating field values. This work primarily focuses on the second category, commonly referred to as relational observables. A recent approach to constructing local observables in GR was introduced by Rovelli (2002b) and formalised by Dittrich (2006, 2007). This approach involves correlating two partial observ- ables—quantities that are individually gauge-variant—to form a gauge-invariant complete observ- able. These complete observables embody a relational notion of locality: instead of…

re (l) ality : Nicola Bamonti1,2 1의 모든 곳에서부터의 견해로부터의 견해, 철학, Scuola Normale Superiore, Piazza Dei Cavalieri, 7, Pisa, 56126, Italy 2department of Philosophy, Geneva, 5 Rue Candolle, 1211 Geneva 4, 1211 Geneva 4, 1211 Work는 두 가지 패러다임을 대조하여 일반 상대성에서 참조 프레임의 개념적 및 수학적 기초를 조사합니다.
Nowhere의 견해는 프레임 표현을 불변의 동등성 클래스의 관점으로 해석하는 반면, 어디에서나 볼 때 각 프레임 표현을 현실 자체로 구성합니다.
이 현실의 개념은 “관계”라고합니다.
이 논문은 실험 실습과 이론을 조정하는 데 중점을 둔 이러한 견해의 철학적이고 실질적인 영향을 비판적으로 조사합니다.
논의의 중심은 온톨로지의 끔찍한 특성을 제공하는 데 어려움이 있습니다.
어디에서나의 견해는 소위 ‘대칭에 대한 정교한 접근법’과 일치하며 이론적 구성의 경험적 근거를 복잡하게 만듭니다.
대조적으로, 모든 곳의 견해는 동등성 클래스의 추상화를 피하는 관계형 온톨로지를 제공합니다.
1 ARXIV : 2412.20520V1 [Physics.Hist-PH] 29 12 월 2024 년 12 월 목차 1 소개 3 소개 3 2 번들 형식에 대한 게이지 관점 7 3 참조 프레임 7 3 FREME 표현은 동등성 클래스의 관점입니다.
관찰 가능성은 이론의 물리적 내용을 변경되지 않은 변형 하에서 변하지 않는 수량을 말합니다.
게이지 변환으로 알려진 이러한 변환은 이론의 기본 형식주의에 대한 수학적 재현을 나타냅니다.
이 논문은 그러한 관측 가능성이 어떻게 정의되고 해석되는지 탐구합니다.
이론이 경험적 타당성을 달성하기 위해서는 게이지 불변 관측 가능성이 측정 가능한 양에 해당해야하며 이론적 예측과 실험 관찰 사이의 비교를 촉진해야합니다.
이 서신은 과학 이론의 수용에 대한 중요한 기준입니다.
우리의 경험의 대부분은 특정 시공간 지점 또는 현지 지역 내에서 로컬로 정의 된 변수를 측정하는 것입니다.
Gary and Giddings (2007)는“우리가 진정으로 관찰 할 수있는 것은 현지화된다 – 우리는 무한대에 접근 할 수 없다”고 강조했다.
GR에서 게이지 대칭은 디스 페이 형성으로 표현된다.
결과적으로, 시공간으로 국부적으로 정의 된 모든 수량은 이러한 변형에 따라 변하지 않으므로 로컬, 게이지 불변 관측 가능성을 구성하는 데 어려움이 생깁니다.
디프트 오모리 비 변동 이론에서 변수는 두 가지 유형으로 광범위하게 분류 될 수 있습니다.
(1) 글로벌 영역에 대한 적분과 같은 전체 시공간에 걸쳐 정의되는 높은 비 국소량 및 (2) 전계 값에 의해 구성되는 관계형 수량과 같은 관계 수량과 같은 두 가지 유형의 수량이 매우 높습니다.
이 작업은 주로 관계형 관찰 가능성이라고하는 두 번째 범주에 중점을 둡니다.
GR에서 국소 관측 가능성을 구성하는 최근의 접근법은 Rovelli (2002b)에 의해 도입되었고 Dittrich (2006, 2007)에 의해 공식화되었다.
이 접근법은 게이지 불가능한 완전한 전망을 형성하기 위해 두 가지 부분 관찰 (개별적으로 측정 변수 인 정량)과 관련이 있습니다.
이 완전한 관찰 가능성은 지역의 관계 적 개념을 구현합니다.